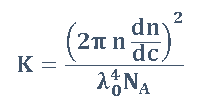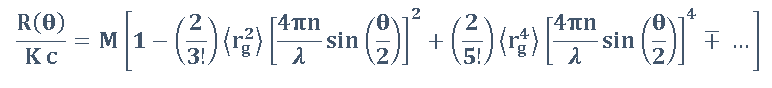Multi-Angle Light Scattering
The basic principle of Static Light Scattering at multiple angles (Multi-Angle Light Scattering) is the same as the principle of Static Light Scattering at one single angle. A beam of polarized light is focused onto the sample molecule and the scattered light is detected with a photo detector. But in Multi-Angle Light Scattering the scattered light is detected at various different angles at the same time. The intensity of the scattered light at each angle is proportional to the molar mass and the concentration of the molecules under investigation. For smaller macromolecules with no angular dependence of the scattered light, the detection of one single angle is sufficient. But when the sample molecules get larger, more and more light is scattered in the forward direction at smaller detection angles. In this case it is absolutely necessary to detect the scattered light at multiple angles at the same time.
In Multi-Angle Light Scattering the basic equation from Static Light Scattering can be used. Multi-Angle Light Scattering in dilute solutions can be expressed by the following equation:
With K
R(θ) = Excess intensity of scattered light at a given angle (θ)
c = Sample concentration
M = Molar mass
A2 = Second viral coefficient
K = Optical constant
n = Solvent refractive index
dn/dc = Refractive index increment
NA = Avogadro’s number
λo = Wavelength in vacuum of the incident laser light
P(θ) = Function of the angular dependent light scattering intensity (form factor)
After the combination of the above scattering equation with the Taylor expansion for the form factor P(θ), the following light scattering equation is obtained:
Using this formula R(θ)/(K*c) can be plotted against sin2(θ/2) and the gyration radius Rg as well as the molar mass M can be calculated. Rg is the slope of the function at angle 0° and M can be derived from the intercept of the curve with the y-axis.

[1] H.C. van de Hulst (1957) “Light Scattering by Small Particles”, Dover Publications Inc., NY
[2] M. Kerker (1969) “The scattering of light and other electromagnetic radiation”, Academic Press, NY